Covered Call is a popular strategy that is often considered as a source of additional income for a long-term equity investor. Writing OTM calls is supposed to be a conservative strategy an average investor can pursue to earn a supplementary gain in excess of the underlying assets return.
However, it turns out that the call overwriting against the major equity indices does not add any meaningful profit to the portfolio because almost all the premium collected from the short calls will eventually be lost in the form of not participating in the growth of the underlying security called-out in the cases of ITM expiration.
This research demonstrates that call options on equity indices are priced quite fairly on average (in the contrast to puts) and usually have expected profit/loss close to zero. Moreover, in the secular bull markets, they are mostly underpriced, and Covered Call imposes a drag on the portfolio performance.
Nevertheless, on a risk-adjusted basis, this strategy can be quite attractive, especially for the ITM calls selling.
In this research, we will analyze the strategies of selling the call options on the SPDR S&P 500 ETF (SPY). It turns out that all other major US equity indices demonstrate very similar results: Dow Jones Industrial Average (DIA), Russell 2000 (IWM), Nasdaq-100 (QQQ), and even equity index of the developed countries excluding US and Canada - MSCI EAFE (EFA).
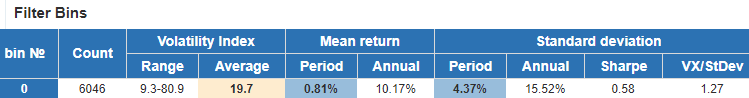
For our analysis, we have taken 4-week (20 trading days) SPY call options and calculate their Fair Values based on the historical underlying return for the 24 years period: from September 1993 to September 2017. The statistics of the underlying returns, without any filtering, is the following:
Here is a comparison of the Fair Values vs Estimated Market Prices of these options:
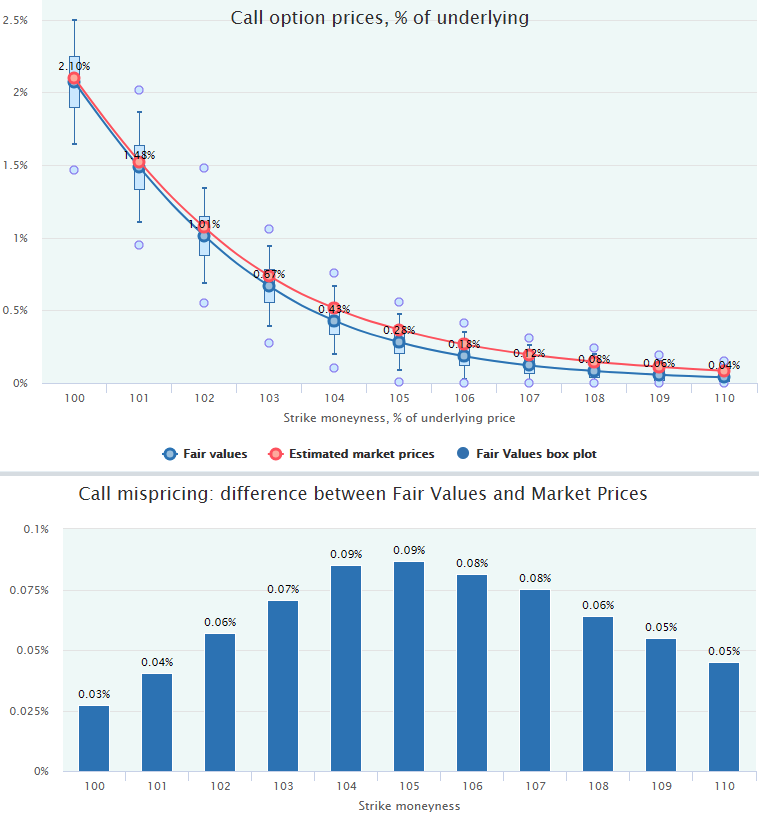
Obviously, both Fair Value and Market Price lines are very close to each other, and, hence, the market mispricing is not meaningful. Some overpricing can be found in the area of 104-106 moneyness, but it is not statistically significant since market prices come through the bodies of the Fair Value box-plots.
Compare that chart with the put options mispricing for the same time interval and underlying security; the staggering difference:
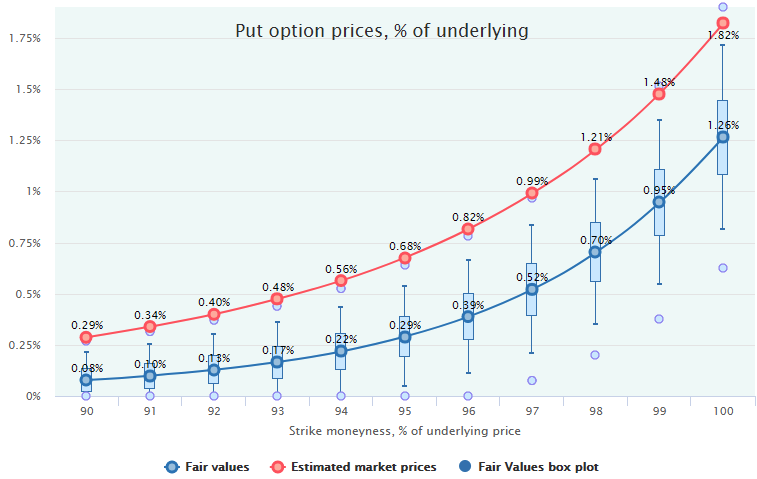
For more about the put options overpricing and its reasons, see the research Are puts really overpriced?
So, the conclusion is that, on average in the long run,
call options are priced almost fair
Therefore, such a "covering" of the long portfolio with a shot call options does not improve the overall profitability. It can even worsen the final performance during the bull markets (see below).
The zero expected profit of the short calls means the following: the options premium collected is equal to the lost income of the called-away underlying in case of in-the-money expirations.
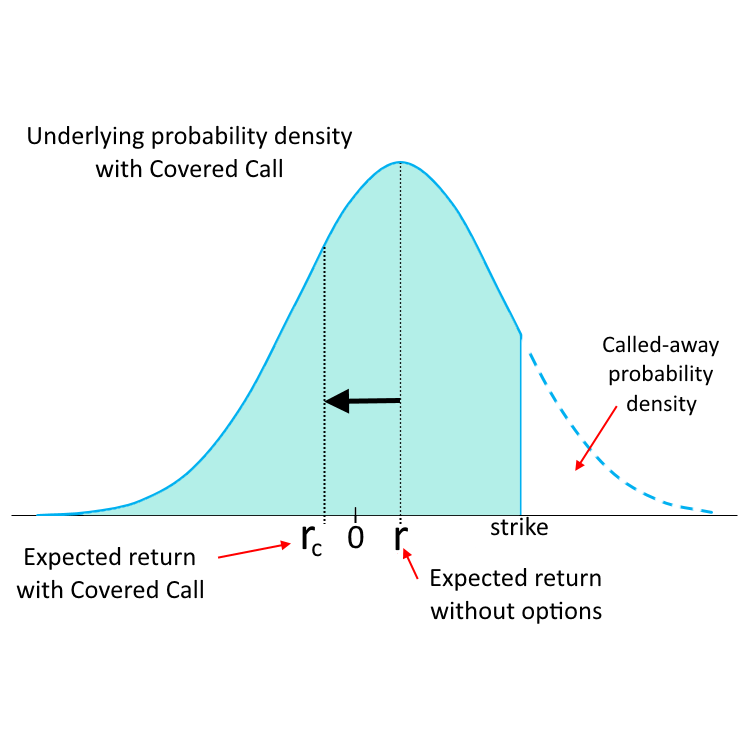
Graphically, it has the following representation. Without any options overlay, the underlying security returns have some probability distribution and an expected return - r - calculated by weighting all possible returns by their probabilities. For equities, this value is usually positive and exceeds the risk-free rate by some equity premium.
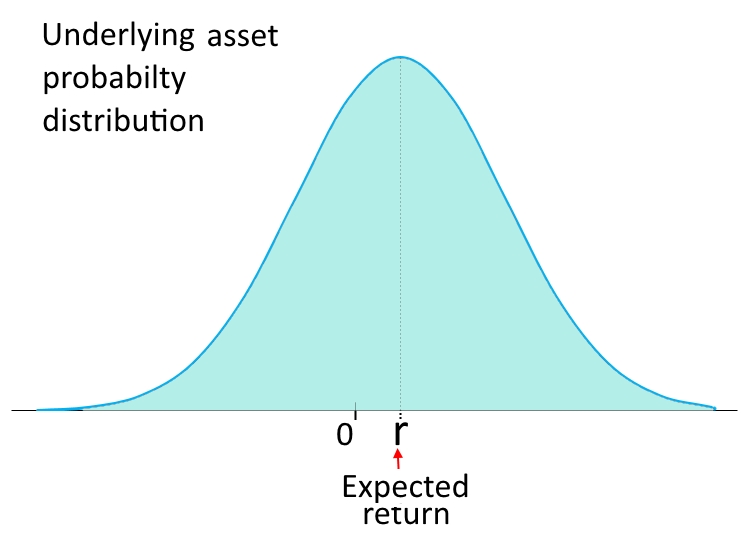
For now, it does not matter what form this probability function has and what the value of r is. We are just discussing the general concept.
If we start to overwrite the underlying asset with short calls, our security will be periodically called away in the cases of the in-the-money expirations (when price exceeds the call strike). That will "cut-off" the right tail of our probability density since we will not possess the underlying security in this zone when it is called out. So, the probability of getting returns in this right tail will be zero for us, whatever that returns will be.
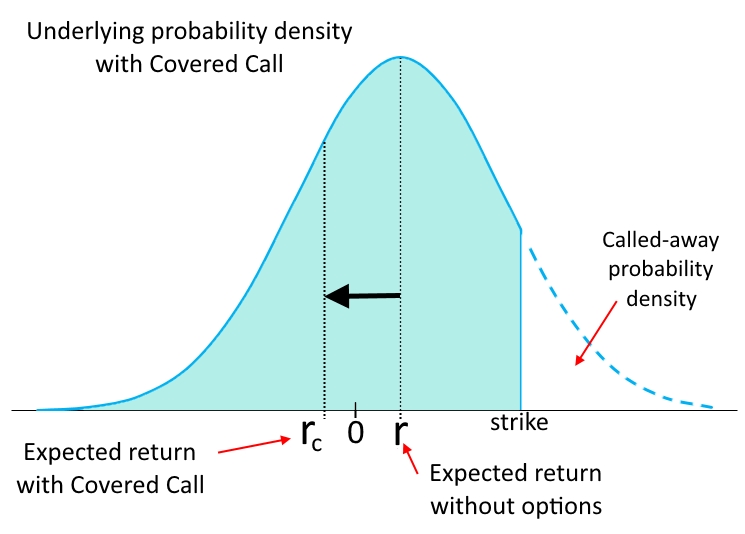
That probability "cutting" leave us with more weight on the negative side and, hence, decrease the expected return of the underlying asset (r) moving it to the left (rc). Therefore, the
negative returns will be less balanced with the positive ones
Theoretically, that loss of the expected return (r - rc) should be compensated by the premium collected from the calls sold. However, it takes place only if the selling of calls has at least non-negative expected profit, i.e., their Fair Values are less or equal to the market prices. That is not always the case for the equity indices, see below.
In other words, the Covered Call will be a profit-adding strategy if the opportunity cost (r - rc) is less than the average premium of calls sold. That opportunity cost is actually the Fair Value of these calls calculated as the ITM expiration probability multiplied by the average option value (payoff) in this case, see the respective methodology article.
So, to make the Covered Call efficient, we need a positive expected profit from the short selling of a call option.
As we have observed at the beginning, on average in the long run, calls on the wide equity indices are fairly priced with the expected profit close to zero. That makes the Covered Call strategy vulnerable to the market regimes: in some markets, it could be a profitable strategy adding a positive value to the underlying portfolio, while in the other, this call overwriting would be detrimental.
Obviously, the major enemy of the short calls are the bull markets. Here is the options mispricing in the last three of them:
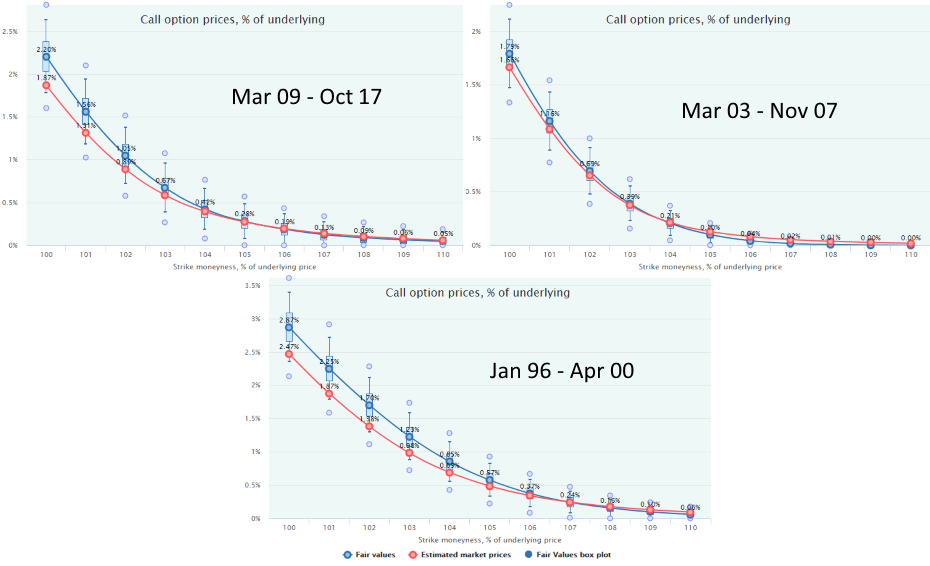
In all these periods, call options have been undervalued by the market. In some of them, statistically significant, especially near-the-money (moneyness close to 100). Therefore, in such periods, the Covered Call strategy has less expected profit than the outright holding of the underlying security. Importantly, these bull markets embraced around 80% of the whole time interval since 1996 making the Covered Call a losing strategy for such a big portion of time.
In the other, bear, markets, the Covered Call strategy is quite efficient due to the downtrending underlying prices and the elevated implied volatility of call options:
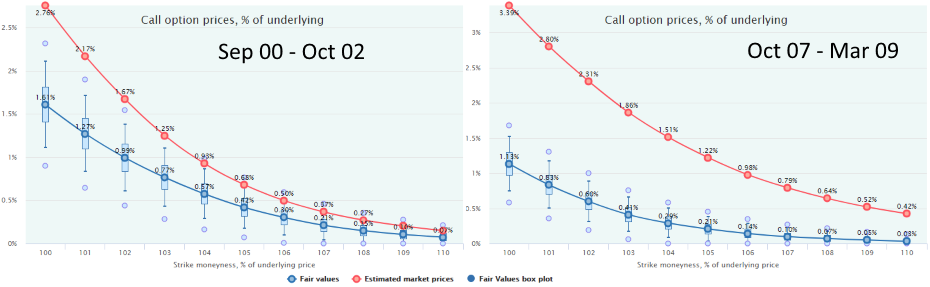
All these findings are consonant with the performance of the CBOE BuyWrite Index (BXM) that emulates the Covered Call strategy on S&P500 with the regular writing of the monthly at-the-money call options.
Here is the performance of that BuyWrite Index against the total return of SPY (including dividends):
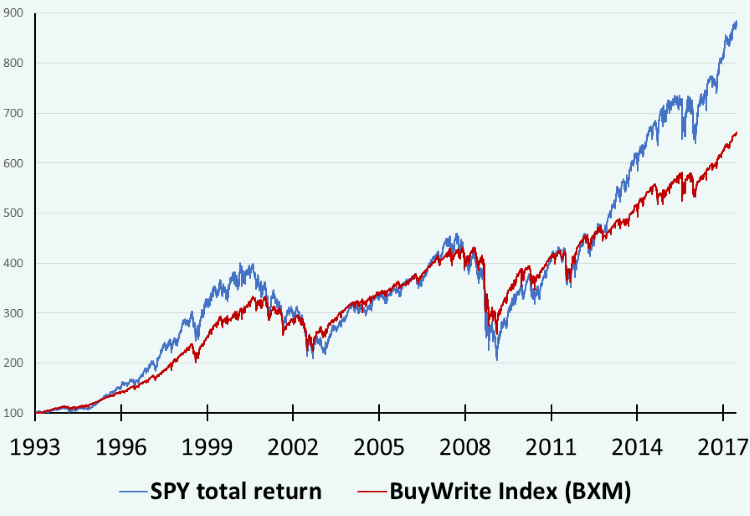
Conclusions are the same as we have got from our analysis here:
It is obvious from the above findings that the advantage of the Covered Call strategy can be found in its lower volatility relative to the simple buying of the underlying security.
The OptionSmile strategy performance metrics for the Covered Calls reveals the fact that it has almost the same average return as the simple buy&hold strategy (remember, calls are valued quite fair) but with less volatility measured by the standard deviation. Even during the underperformance in the bull markets, such a strategy has substantially less volatility.
Here are the underlying returns statistics and some key Covered Call performance metrics for the whole 24-year period (September 1993 - September 2017), 4-week (20 trading days) calls - without filtering:
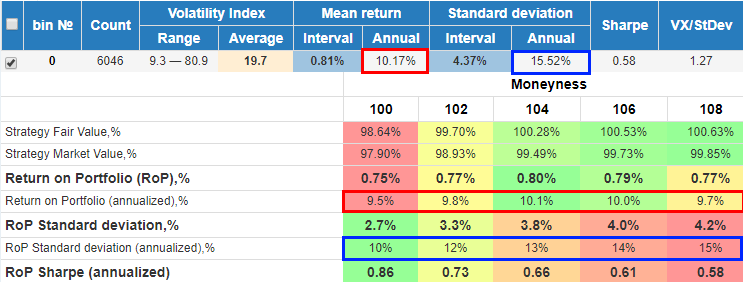
Here we see that average Return on Portfolio (100% position sizing) is not far from the total return of the underlying security - somewhere around 10% annually. That confirms that the short call does not add much to the total expected profitability, see the chart at the beginning.
However, the volatility measured by the standard deviation of these returns is much less than the underlying returns have. For example, with the call moneyness of 100, it is 10% against 15.5% of the underlying, which leads to a higher Sharpe ratio (0.86 vs 0.58). This effect fades with the moving farther out-of-the-money due to the less option premium.
For the bull markets, when the Covered Call substantially underperforms, that pattern remains intact. Here are the same metrics for three recent bull markets (Sep 93 - Apr 00, Mar 03 - Nov 07, Mar 09 - Sep 17):
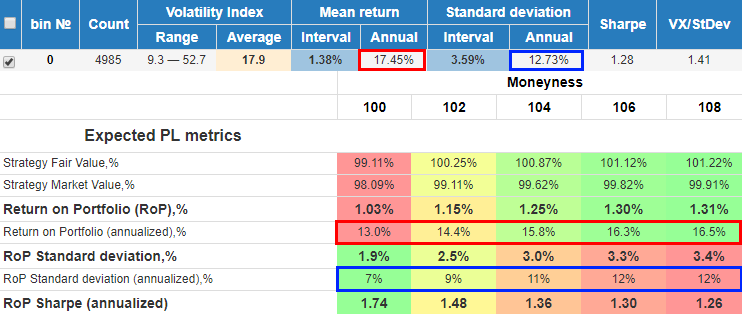
Here we have significantly less profitability of Covered Call near-the-money, but with substantially less standard deviation. That leads to much higher Sharp Ratio of this strategy.
So, the major conclusion is that the Covered Call strategy has
less profitability but better risk-adjusted performance
than an outright buy&hold strategy, if we measure risk by the standard deviation.
As an obvious outcome, it would seem to be a good strategy to leverage the Covered Call proportionally to its volatility difference with SPY. That would result in the supreme performance: more income with the same volatility.
Although it is a viable idea, some words of caution are necessary. Actually, the standard deviation reduction is going with the sacrificing of positive returns (see the discussion above) while negative drawdowns still remain in the distributions (though, somewhat muted by the calls' premium collected).
Here is the Profit/Loss histogram of the 4-week Covered Call strategy with 100 moneyness in comparison with the respective SPY returns distribution (24 years, without filtering):
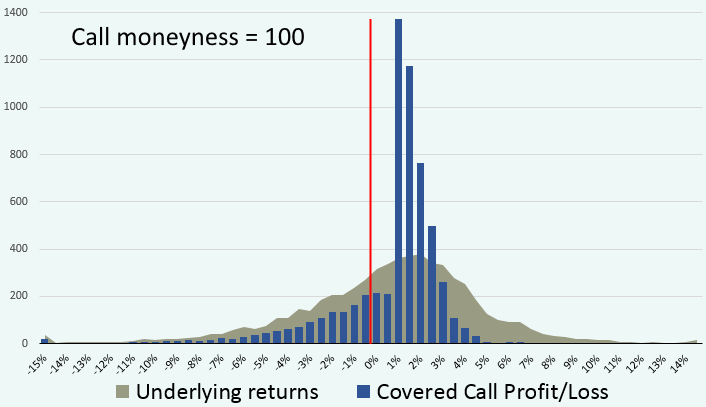
Evidently, the Covered Call strategy "pulls" some probability density from the tails to the center of the distribution. Mostly, it cuts off the right, positive returns - the ITM expirations. Negative tail remains as long as in the underlying returns distribution, but they are partially compensated with the call premiums received.
Interestingly enough, this picture significantly changes when we move the call strike to either OTM or ITM area:
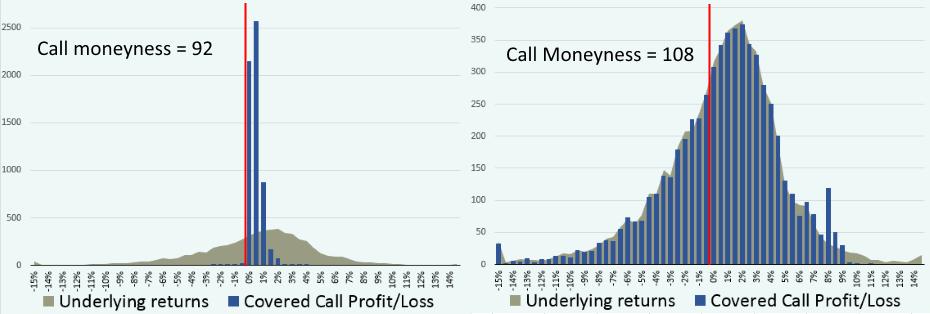
ITM Covered Call (moneyness 92) has gathered almost the whole probability from both tails to the center, while the ITM strategy (moneyness 108) almost fully resembles the underlying distribution, except for the father right tail.
For more about the superior performance of Covered Call strategy, even in Bull Markets, see the respective research.